Exponential dispersion model
Exponential dispersion models are statistical models in which the probability distribution is of a special form.[1][2] This class of models represents a generalisation of the exponential family of models which themselves play an important role in statistical theory because they have a special structure which enables deductions to be made about appropriate statistical inference.
Definition
Exponential dispersion models are a generalisation of the natural exponential family: these have a probability density function which, for a multivariate model, can be written as
where the parameter 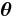 has the same dimension as the observation variable
has the same dimension as the observation variable  . The generalisation includes an extra scalar "index parameter",
. The generalisation includes an extra scalar "index parameter",  , and has density function of the form[2]
, and has density function of the form[2]
The terminology "dispersion parameter" is used for 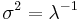 , while
, while 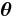 is the "natural parameter" (also known as "canonical parameter").
is the "natural parameter" (also known as "canonical parameter").
References
- ^ Marriott, P. (2005) "Local Mixtures and Exponential Dispersion Models" pdf
- ^ a b Jørgensen, B. (1987). Exponential dispersion models (with discussion). Journal of the Royal Statistical Society, Series B, 49 (2), 127–162.
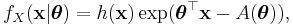
![f_X(\mathbf{x}|\lambda,\boldsymbol{\theta}) = h(\lambda,\mathbf{x}) \exp (\lambda [\boldsymbol\theta^\top \mathbf{x} - A(\boldsymbol\theta)] ) \,\! .](/2012-wikipedia_en_all_nopic_01_2012/I/67b5f53309b74587930e0059920e15c1.png)